Page 27 - El Modelo de Regresión Lineal

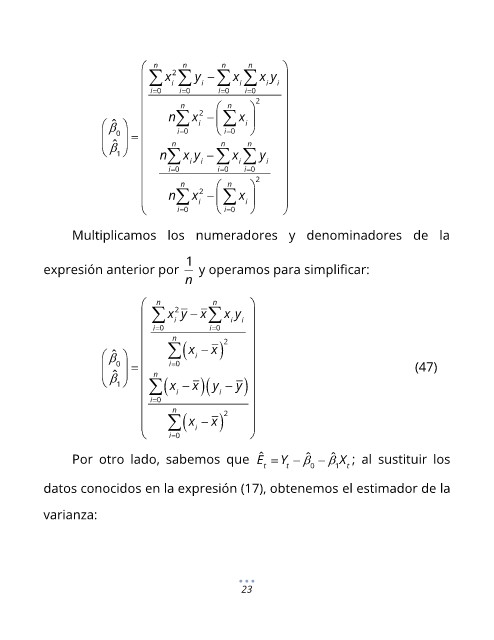
 n 2 n n n 
i  
 x i  y − x i x y i 
i
 i= 0 i= 0 i= 0 i= 0 
 n  n  2 
2
 n x −   x i  
ˆ 
  =  i i   
 0  =  i 0 = 0 
ˆ 

n
1 
i  
   n  x y − n x n y 
 i= 0 i i= 0 i i= 0 i 
 n  n  2 
 n x −   x i  
2
 i 
 i = 0 i  = 0  
Multiplicamos los numeradores y denominadores de la

1
expresión anterior por y operamos para simplificar:
n

 n 2 n 
 x y − x  x y i  
i
i
 i = 0 i = 0 
 n (  x ) 2 
   x − 
ˆ 
i
  ˆ  0  =  n i= 0  (47)
1 
   (  x − x )( y − y ) 
 i i 
 i= 0 n 
 (  x − x ) 2 
 i 
 i= 0 

ˆ
Por otro lado, sabemos que E = Y −  ˆ  − ˆ X ; al sustituir los
t t 0 1 t

datos conocidos en la expresión (17), obtenemos el estimador de la

varianza:

23