Page 15 - La matriz inversa

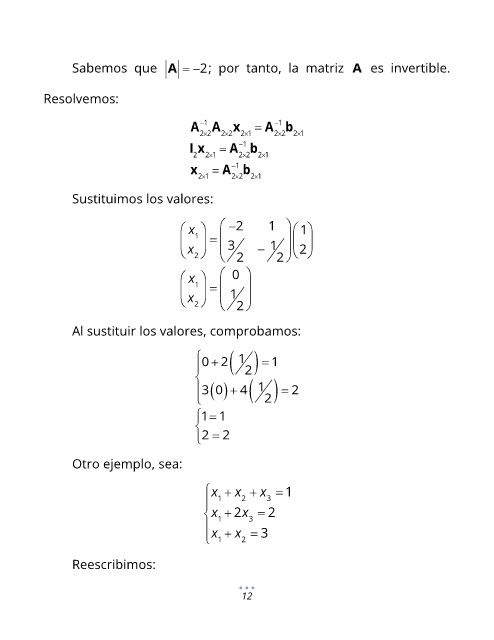
Sabemos que A = − 2; por tanto, la matriz A es invertible.

Resolvemos:

A A x 2 1 = A b 
−
1
−
1
2 2
2 2
2 2 2 1




1
I x = A b
−



2 2 1 2 2 2 1
1
x 2 1 = A b 
−
2 2 2 1


Sustituimos los valores:
 x   2 1  −  1
 1  =  3 1   
 x 2    2 − 2    2 
 x   0 
 1  =  1 
 x 2    2  
Al sustituir los valores, comprobamos:

 0 2 ( ) = 1
1
+
 2

1
3 0 +  ( ) 4 ( ) = 2
 2
 1 1
=

 2 = 2

Otro ejemplo, sea:

 x + x + x = 1
 1 2 3
 x + 1 2x = 3 2
 3
 x + 1 x = 2

Reescribimos:

12